In order to be a successful math student, you have to persevere through various problems. This is a skill that can be taught and must be practiced.
(Noelle recently presented an amazing math training – Practical Problem Solving Strategies – this summer, and I was truly amazed at just how much I learned. I will be breaking down the training into 3 blog posts over the course of this month, so if you missed the training, be sure to check back here for more updates.)
If you want to learn more, check out this book, Mathematize It!, that covers the topic of teaching how to solve word problems in much more detail.
Be sure to read Part 1, Three Word Problem Types to Teach, and grab our freebie below!

Here are the 3 steps to solving word problems:
1. Restate the Problem Situation
Let’s use this word problem about Pedro as our example:
(Check back to last week’s blog post to understand why this scenario falls in the action category.)
By restating the problem, we want students to avoid seeing phrases like “leftover” and decide immediately that they must subtract. We want students to focus on the action taking place. Here is an example of restating the problem. You could have students do this verbally (think, pair, share style) or write down bullet points. Notice that there are no numbers present.
- Pedro makes a pitcher of lemonade.
- He pours some for his friends and now he has some left over.
- How much lemonade did he start with?
2. Represent the Problem Situation
There are numerous ways to represent a problem: draw a picture, create a diagram or model, use manipulatives, or write an equation (don’t think numbers and variables; it can be something like “Pedro’s pitcher = leftovers + what he poured”).
The primary goal of this stage is that representations give students that “operational sense” they need in order to write an equation. We want them to know what to do next.
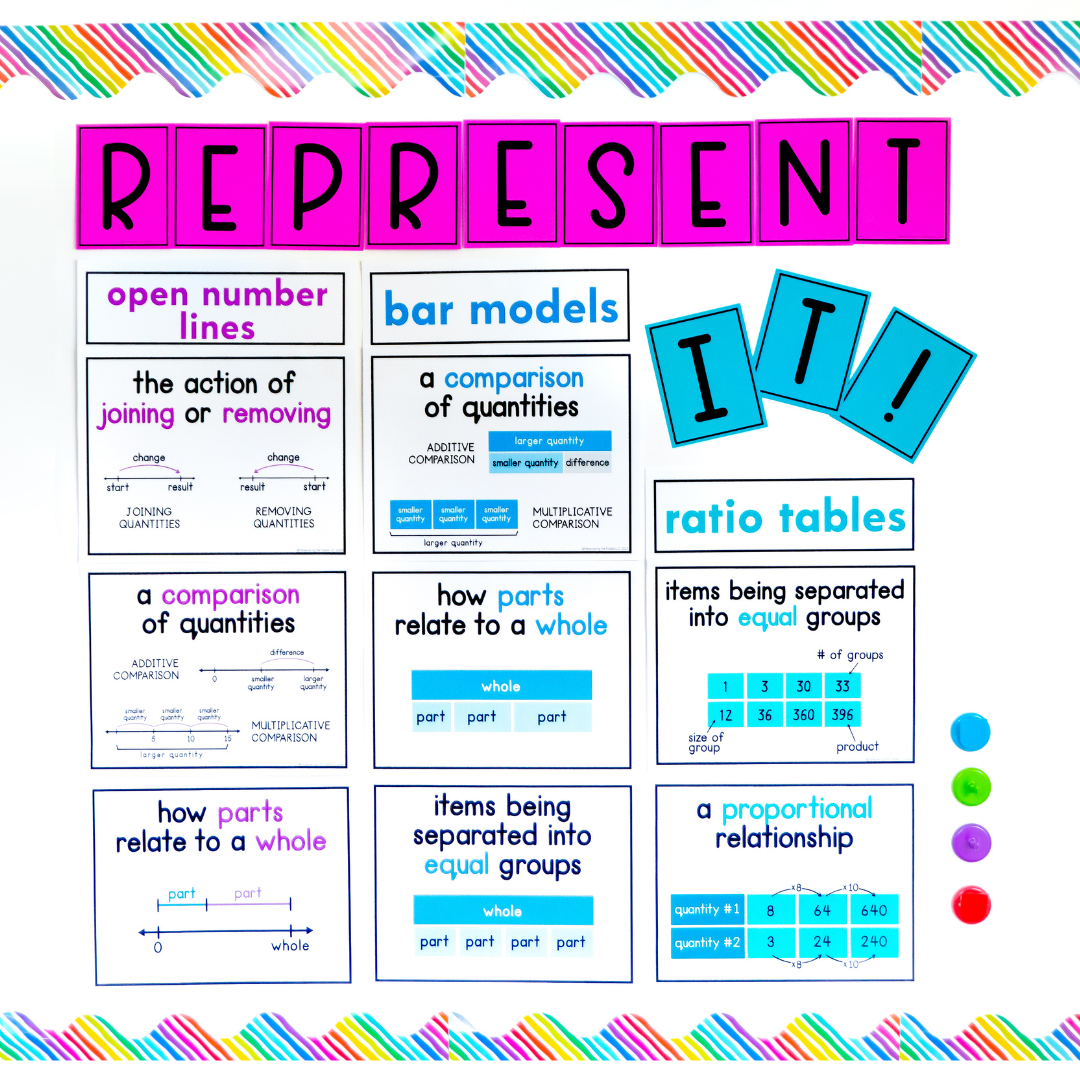
The 3 diagrams we use most in middle school math are open number lines, bar models, and ratio tables. For this problem, I recommend using an open number line.
You can also make the number line a vertical number line. Liquid in a pitcher as a vertical number line might make more sense visually to students.
There was a starting amount of lemonade in the pitcher, but we actually don’t know what that is, so that is our unknown value that we will represent with the variable x on the number line.
Then Pedro pours 34 ounces of lemonade for his friends, here is our action or change, so we will represent that with a jump on the number line. Since we know our action describes removing lemonade from the pitcher, our jump will point down.
Now he looks at the pitcher and there are 20 ounces of lemonade left, this is the resulting value. The open number line allows us to identify what each value in the situation represents, and now we know that our unknown value was the starting amount.
To finish out our lemonade problem, let’s use our representation to write an equation.
We have a starting amount, x, then we will subtract the amount he poured, 34, and that is equal to the amount remaining in the pitcher, 20.
Notice how the equation has subtraction in it, (which makes sense because the situation describes removing a quantity) but the operation we will perform to find the solution is addition. This is what we mean by developing operational sense for a situation.
The number line also helps students see that the solution needs to be a value greater than 20, since x is higher on the vertical number line.
These representations can be so powerful as we help transition students from the concrete to the abstract!
Be sure to grab our free Problem Solving Posters that have examples of all of these representations.
3. Solve and Reflect
When we think about problem solving, we often think about the end goal being to find the solution, which of course is important.
But in order to grow our students as problem solvers, it’s important to also take time for individual and classroom reflection. Reflection is such an important part of making meaning. If students can construct mathematical arguments to justify their solutions, you know they fully understood the problem.
After students have worked through a word problem, encourage them to share the different models or equations that they came up with, and have them explain the operations they used to solve a problem. Students will learn from each other as they are exposed to different ways of thinking about the same problem.
P.S. Check out these related posts: Math Problem Solving Strategies and How to Teach Word Problems and Problem Solving